Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreExample 2 if x = 10 and y is 4 (10 4) 2 = 10 2 2·10·4 4 2 = 100 80 16 = 36 The opposite is also true 25 a 4a 2 = 5 2 2·2·5 (2a) 2 = (5 2a) 2 Consequences of the above formulasJul 08, 17 · Q29 If x y = 4, then find the value of x 3 y 3 12xy – 64 Q30 Without actually calculating the cubes, find the values of (i) (27) 3 (–17) 3 (–10)3 (ii) (–28) 3 (15) 3 (13) 3 Q31 Using suitable identify, find the value of 86 × 86

Expand Each Of The Following Using Suitable Identities 2x 3y 2z 2
Expand using suitable identity x + 2 y + 4 z square
Expand using suitable identity x + 2 y + 4 z square-On the left diagram above, the red rectangle is inscribed in a larger outside rectangle On the right, in the same outside rectangle, two blue rectangles are formed by the perpendicular lines arising from 2 adjacent vertices of the red rectangleCorrect answer to the question We are trying to factorize (8x³−y³−z³−6xyz) Using a suitable algebric identity This is what we get (2xPy−Qz)(4x²y²z²Rxy−yz2zx) Find the missing values P= Q= R= brainsanswersincom



Algebraic Identities Of Polynomials A Plus Topper
Separate f and z into real and imaginary parts f(z) = u(x,y)iv(x,y) where z = x iy and u, v are real functions Suppose that f is differentiable at z We can take δz in any direction;Jan 30, 18 · Using suitable identity, evaluate the following (i) 103 3 (ii) 101 x 102 (iii) 999 2 Firstly adjust the given number into two number such that one is a multiple of 10 and use the proper identityA Answers, Solution Outlines and Comments to Exercises Chapter 1 Preliminary Test (page 3) 1 p 7 c2 = a2 b2 2abcosC (5 marks) 2 x 4=3 y 16 = 1 Verify that the point is on the curve Find slope dy dx = 12 (at that point) and the tangent y8 = 12(x2)
Anupam Khosla has stated it all, I will slightly detail it and add one more point in the end Let me first remind what is math(abc)^2 /math math(abc)^2 = a^2 b^2 c^2 2(abbcac)/math math(abc)^3 = (abc)^2 (abc)/math Thu(4) This modulus is equivalent to the euclidean norm of the 2D vector (x;y), hence it obviously satisfy the triangle inequality jz 1 z 2j jz 1j jz 2j However we can verify that jz 1z 2j= jz 1jjz 2j Division z 1 z 2 = z 1z 2 z 2z 2 = (x 1 iy 1)(x 2 iy 2) x2 2 y2 2 = x 1x 2 y 1y 2 x2 2 y2 2NCERT Solutions Class 8 Maths Chapter 14 Factorisation PDF are provided here for free These NCERT class 8 maths solutions for Chapter 14 Factorisation can
first take it to be real, δz = δx Then f0(z) = lim δx→0 f(z δx)−f(z) δx = lim δx→0 u(xδx,y)iv(xδx,y)−u(x,y)−iv(x,y) δx = lim δx386 Chapter 15 Multiple Integration c y 1 y 2 y 3 d a x 1 x 2 x 3 x 4 x 5 b ∆x ∆y Figure 1511 A rectangular subdivision of a,b×c,d Using sigma notation,Jan 19, 21 · using identity evaluate 107 Mathematics TopperLearningcom o5uhf4ybb Using suitable identity, evaluate the following Share 4 Get a free home demo of LearnNext Evaluate each of the following using identities (i) (399)^2 (ii) (098)^2 (iii) 991 x 1009 (iv) 117 x asked Feb 11 in Algebraic Expressions by ShasiRaj ( 624k points) algebraic identities Expert Answer



Expand Using Identity 2x 3y 3
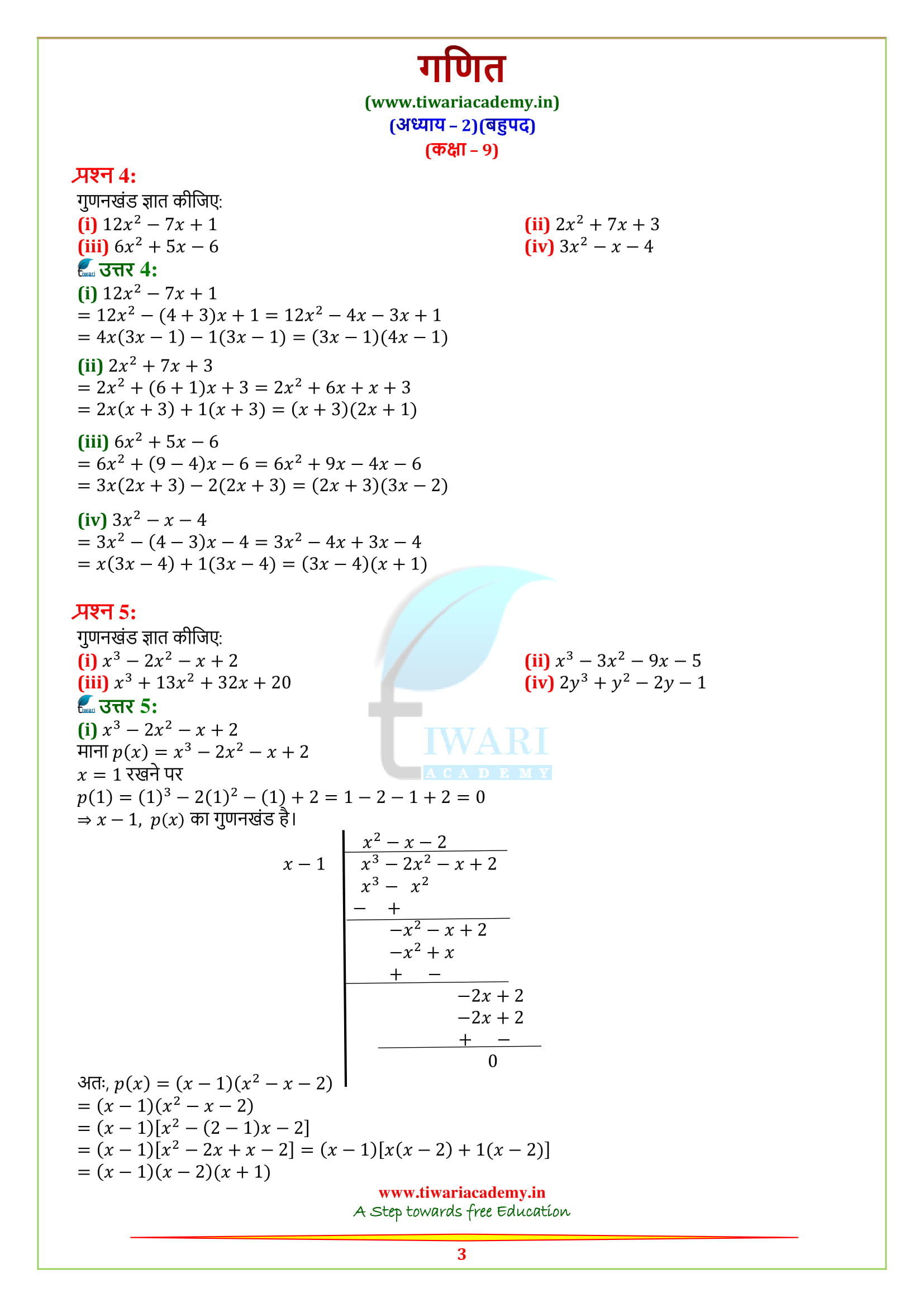


Ncert Solutions For Class 9 Maths Chapter 2 Polynomials In Pdf
Z 4 z 3 2 z 2 4 z 8 = 0 b) Find all the roots root of this equation P(z) = z 4 a z 3 b z 2 c z d is a polynomial where a, b, c and d are real numbers Find a, b, c and d if two zeros of polynomial P are the following complex numbers 2 i and 1 i Solutions to the Above QuestionsA method and system of processing seismic data includes acquiring threecomponent seismic data and combining horizontal components of the threecomponent seismic data to obtain a merged horizontal component Frequency spectra are determined for the acquired threecomponent seismic data A ratio of a vertical component of the seismic data to the merged horizontalThe square function is related to distance through the Pythagorean theorem and its generalization, the parallelogram law Euclidean distance is not a smooth function the threedimensional graph of distance from a fixed point forms a cone, with a nonsmooth point at the tip of the coneHowever, the square of the distance (denoted d 2 or r 2), which has a paraboloid as its graph, is a smooth
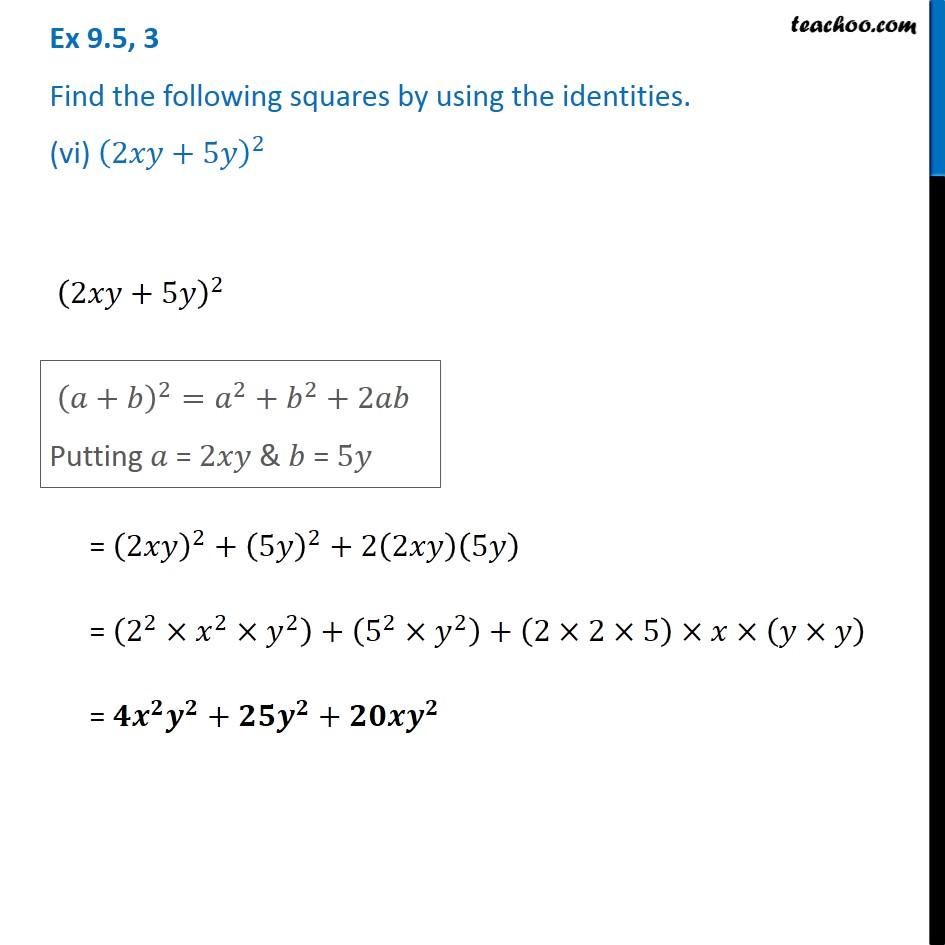


Ex 9 5 3 Find The Squares By Using Identities I B 7 2



Ex 2 5 4 Expand Each Of The Following Using Suitable
However, 1 is not between 0 and 3, and indeed –10mod 3 2, since –10 = –4 * 3 2 Some languages (C, Java) have only a remainder operation, some languages (Pascal, Modula2) have only a modulo operation, and some languages (Ada, Scheme, Haskell) have both (b) Indeed, the above differences can cause the results of the gcd to differ by aDec 23, 17 · Expand the following (2xyz)the whole raised to 2 Share with your friends Share 0∇2f(x,y,z) = 02∇(xy z)·∇(x−2z) = 2(ij k)·(i−2k) = 2(10−2) = −2 This example may be checked by expanding (x y z)(x − 2z) and directly calculating the Laplacian Exercise 4 Use this rule to calculate the Laplacian of the scalar fields given below (click on



Expand Each Of The Following Using Suitable Identity I X 2y 4z Ii 2x Y Z Iii Brainly In


Laminin 511 E8 Promotes Efficient In Vitro Expansion Of Human Limbal Melanocytes Scientific Reports
Parabola is x2(y−5) 2 As 16y = x , the distancesquared isf(y) = 16y(y−5)2 As df/dy = 2y6, there is only one critical point, at y = −3;May 01, · We present a new variant of Gronwall's lemma which improves the variant of Gronwall's lemma in Liu (14) Based on this new variant of Gronwall's lemma, we prove the existence and uniqueness of almost automorphic solution in distribution for some stochastic differential equations driven by Levy noise, which improves and generalizes the results inJan 24, 18 · You can't, really While it looks like you can just cancel out the squares with the square root, this only works if you have one square within the radical Since we're adding two together, it's slightly different There's no way to factor x^2y^2 to get a perfect square The only real way to simplify this expression is by using math that's at a higher than algebra level, so for


Be Awesome In Ggplot2 A Practical Guide To Be Highly Effective R Software And Data Visualization Easy Guides Wiki Sthda


Samacheer Kalvi 8th Maths Solutions Term 1 Chapter 3 Algebra Intext Questions Samacheer Kalvi
Factor the trinomial 9x 2 3x 2 Solution To factor the above trinomial, we need to write it in the form 9x 2 3x 2 = (ax m)(bx n) Expand the product on the right above 9x 2 3x 2 = abx 2 x(mb na) mn For the polynomial on the left to be equal to the polynomial on the right we need to have equal corresponding coefficientsZz = x2 y2;If you use these options you will find that (x*y*z)has(x*y, has_optionsalgebraic) indeed returns true Besides matching some of the factors of a product also powers match as often as is possible without getting negative exponents For example (x^5*y^2*z)subs(x^2*y^2==c, subs_optionsalgebraic) will return x*c^2*z



Expand 2x 3y 2z 2 Brainly In



The Binomial Theorem Explained With A Special Splash Of Pascal S By Brett Berry Math Hacks Medium
A set X is a subset of set Y (Written as X ⊆ Y) if every element of X is an element of set Y Example 1 − Let, X = {1,2,3,4,5,6} and Y = {1,2} Here set Y is a subset of set X as all the elements of set Y is in set X Hence, we can write Y⊆X Example 2 − Let, X = {1,2,3} and Y = {1,2,3} Here set Y is a subset (not a proper subset) ofDz = 0 since the degree of z4 1 is 2 more than the degree of z2 1 The singularities are at the solutions of the equation z4 1 = 0, that is z = e πı/4,z = e3πı/4,z = e5,z = e7πı/4 The only singularities in the upper half plane are z = eπı/4,z = e3πı/4, and they are simple poles It follows that Z ∞ −∞ x2 1 x 41 dxWithout calculation, decide if each of the integrals below are positive, negative, or zero Let W be the solid bounded by z = {eq}\sqrt { x^2 y^2 } {/eq} and z = 2



Expand And Simplify Binomial Squares 2x 3y 2 Youtube



Ncert Exemplar Class 8 Maths Chapter 7 Algebraic Expressions Identities And Factorisation Learn Cbse
Download free PDF of best NCERT Solutions , Class 9, Math, CBSE Polynomials All NCERT textbook questions have been solved by our expert teachers You can also get free sample papers, Notes, Important QuestionsSuitable identities is (x y z) 3 = x 3 y 3 z 3 2 x y 2 y z 2 x z Therefore, (− 5 x) 2 (4 y) 2 (2 z) 2 2 Factorise 1 3 b 3 8 c 3 − 6 b c using the identity a 3 b 3 c 3In mathematics and physics, Laplace's equation is a secondorder partial differential equation named after PierreSimon Laplace who first studied its properties This is often written as = =, where = = is the Laplace operator, is the divergence operator (also symbolized "div"), is the gradient operator (also symbolized "grad"), and (,,) is a twicedifferentiable realvalued function


Expand Each Of The Following Using Suitable Identities I X 2y 4z 2 Ii 2x Y Z 2 Sarthaks Econnect Largest Online Education Community



Ncert Exemplar Class 8 Maths Chapter 7 Algebraic Expressions Identities And Factorisation Learn Cbse
Expanding Logarithms When you are asked to expand log expressions, your goal is to express a single logarithmic expression into many individual parts or componentsThis process is the exact opposite of condensing logarithms because you compress a bunch of log expressions into a simpler one The best way to illustrate this concept is to show a lot of examplesProblem 2 Compute the derivative of cos(sin(3x2 2xlnx)) Note that if you can do this derivativeClick here👆to get an answer to your question ️ Expand each of the following, using suitable identities (2x y z)^2 Join / Login > 8th > Maths > Squares and Square Roots > Finding Square of a Number Find the square of the following numbers using the identity (a


Cbse 9 Math Ncert Solutions
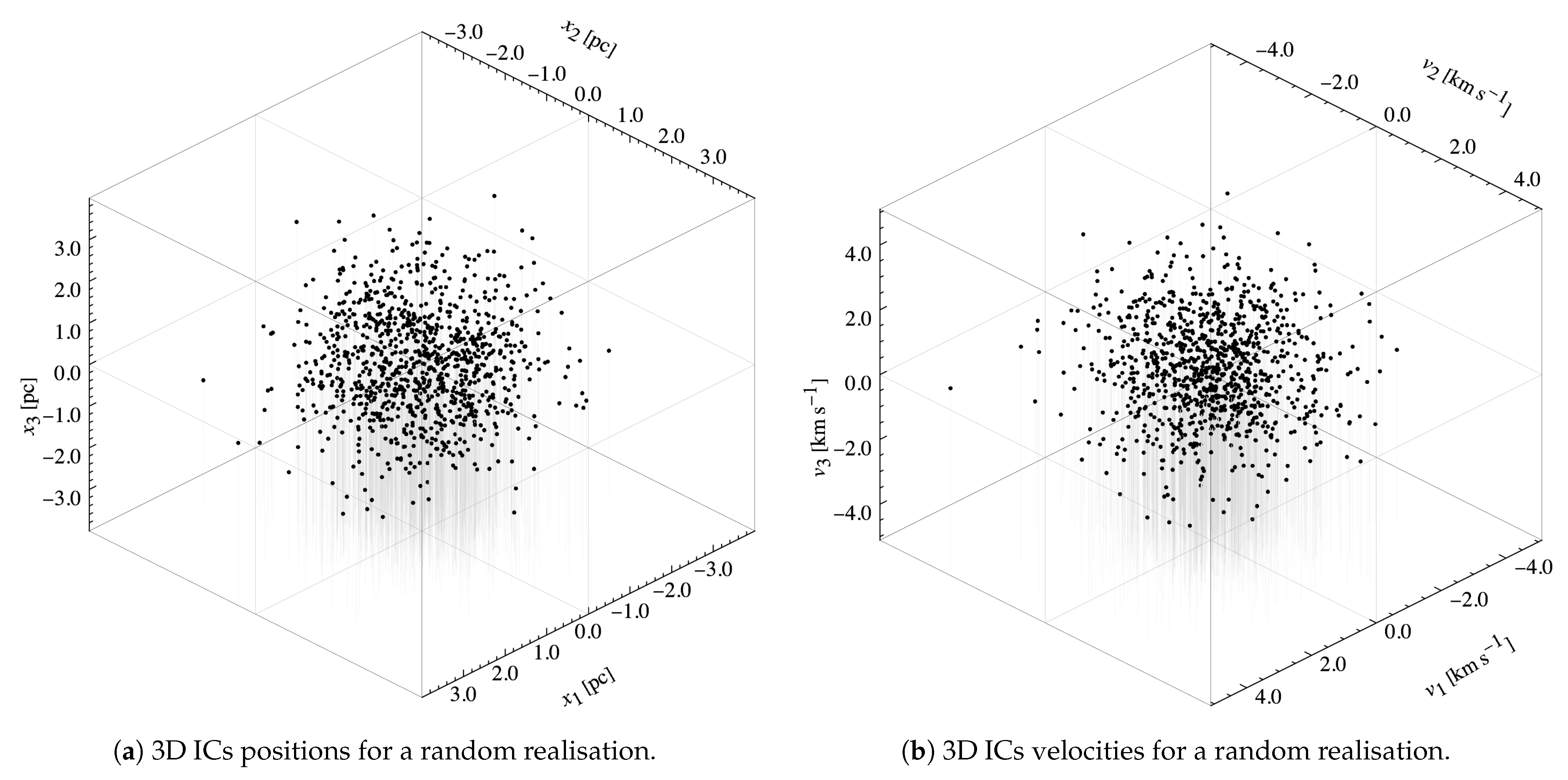


Universe Free Full Text The Clustering Dynamics Of Primordial Black Boles In N Body Simulations Html
Surface integrals Find the area of the portion of the cone x^2y^2=z^2 above the xy plane and inside the cylinder x^2y^2=ax Surface integrals Find the area of the portion of the cone x 2 y 2 = z 2 above the x y plane and inside the cylinder x 2 y 2 = a xWrite the coefficient of y in the expansion of (5y) to the whole squareJan 01, 05 · AMS subject classifications 41A60, 33C65 1 Introduction Asymptotic analysis is a useful mathematical tool which provides analytical insight and numerical information about the solutions of complicated problems in applied mathematics, engineering, physics and many other sciences, which require a mathematical framework for describing and modeling scientific problems



Polynomial Chaos Based Bayesian Approach For State And Parameter Estimations Journal Of Guidance Control And Dynamics



Expand Each Of The Following Using Suitable Identities I
2 1 (tan ) 1 = d x dx x 4 –1 2 1 (cot ) 1 d x dx x − = 5 –1 2 1 (sec ) , 1 1 d x x dx x x = > − 6 –1 2 1 (cosec ) , 1 1 d x x dx x x − = > − 5111 Exponential and logarithmic functions (i) The exponential function with positive base b > 1 is the function y = f (x) =Academiaedu is a platform for academics to share research papersMar 19, 21 · Figure \(\PageIndex{4}\) The Three Equivalent 2p Orbitals of the Hydrogen Atom The surfaces shown enclose 90% of the total electron probability fo\(r\) the 2p x, 2p y, and 2p z orbitals Each orbital is oriented along the axis indicated by the subscript and a nodal plane that is perpendicula\(r\) to that axis bisects each 2p orbital
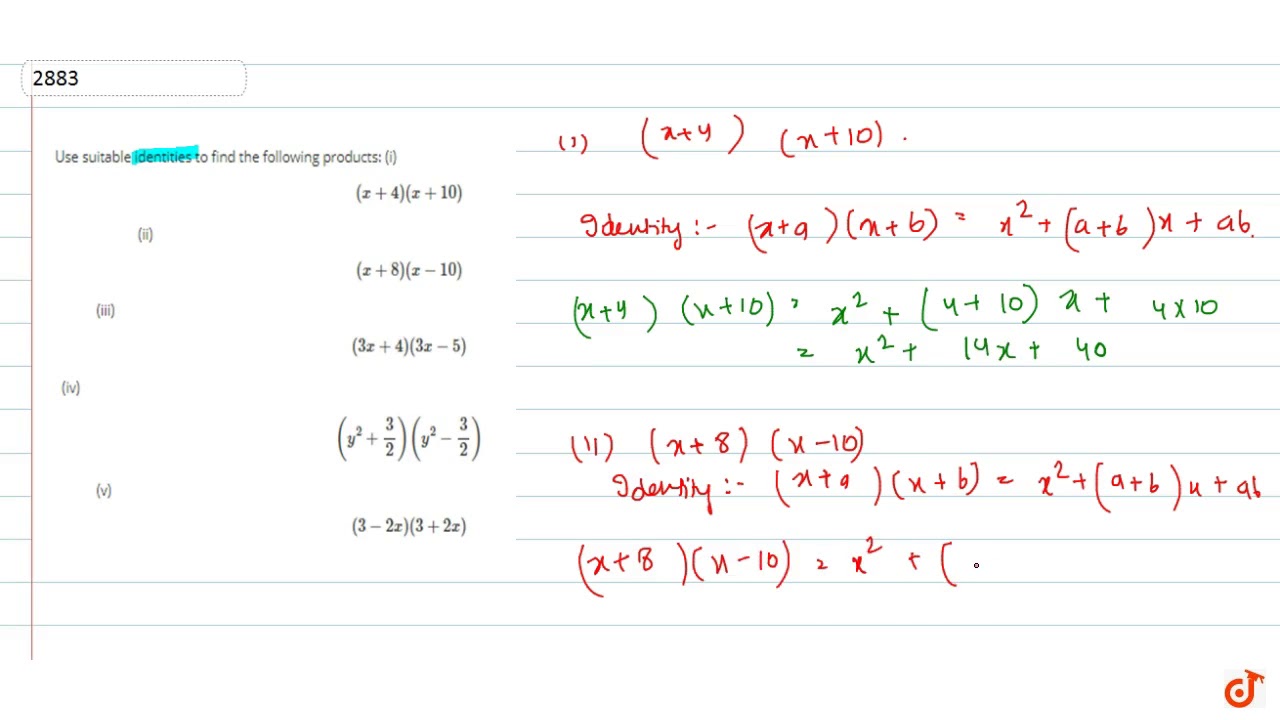


Use Suitable Identities To Find The Following Products I X 4 X 10 Ii X 8 X 10 Ii Youtube



Algebraic Identities Of Polynomials A Plus Topper
However, there is no x such that (x,−3) is on the parabola Thus there is no shortest distance!Y = 225 /065 = since x y = 50, then you get y = x = 50 minus y = those values of x and y should be your answers to confirm, replace x and y in the two original equations to get x y = 50 becomes which becomes 50 = 50 which is trueJul 14, 18 · Find an answer to your question expand by using identity (2x y z)^2 shubham5616 shubham5616 Math Secondary School answered Expand by using identity (2x y z)^2 2 See answers gaurav13c gaurav13c MarkAsBrainliest MarkAsBrainliest We


Perform Symbolic Computations Matlab Simulink


X 2 Y 2 4z 2 2xy 4yz 4xzdivide By X Y 2z Mathematics Topperlearning Com Yqcsiqrr
A method and system for processing three component seismic data includes determining a covariance data matrix from three component seismic data for each of a plurality of time periods to obtain eigenvectors and eigenvalues One or more seismic attributes are calculated from the eigenvectors and eigenvalues for each of a plurality of time periods(iii) y × 2x 2 y × 2xy 2 =(1 × 2 × 2 )( x × x 2 × x × y × y × y 2) = 4x 4 y 4 (iv) a x 2b x 3c = (1 × 2 × 3 ) (a × b × c) = 6abc 5 Obtain the product of (i) xy, yz, zx (ii) a, – a 2 , a 3 (iii) 2, 4y, 8y 2 , 16y 3 (iv) a, 2b, 3c, 6abc (v) m, – mn, mnp Solution (i) xy × yz × zx = x 2 y 2 z 2 (ii) a × – a 2 × a 3 = – a 6November 15 In class, we looked at some additional tricks, such as long division, for computing Laurent series, and we talked about the notion of the residue of an analytic function at a singular point Here is the assignment for next time (not to hand in, but be prepared for a quiz)


Cbse 9 Math Ncert Solutions



Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes
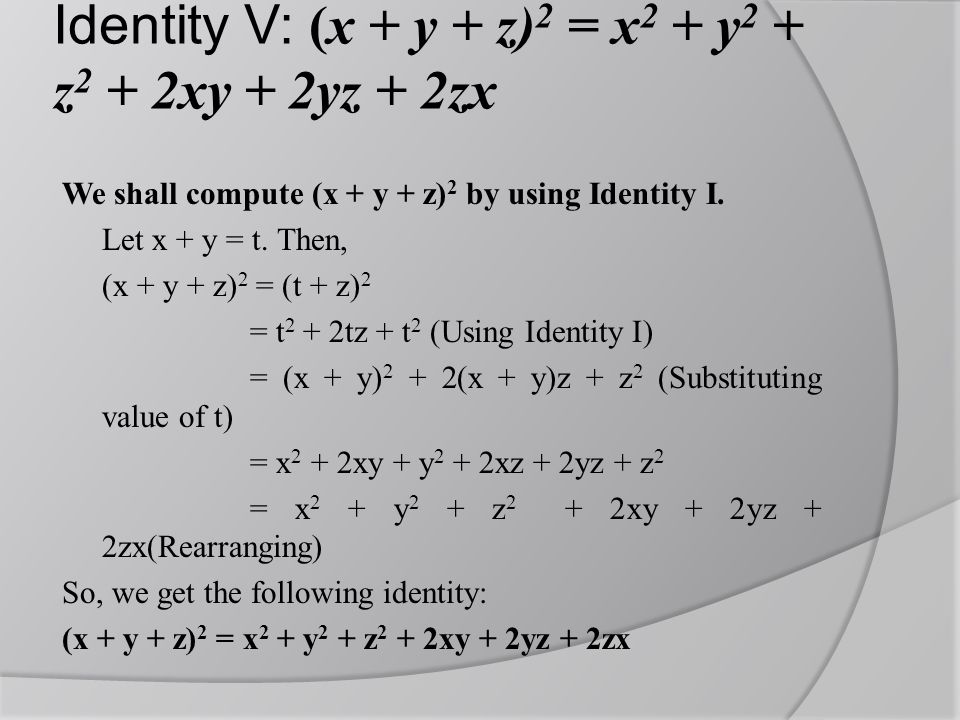
Dec 08, 16 · Example 19 Write (3a 4b 5c)2 in expanded form (3a 4b 5c)2 Using (x y z)2 = x2 y2 z2 2xy 2yz 2zx Putting x = 3a, y = 4b, z =



Mathematics Mathematics For Economics An



Polynomials Ppt Video Online Download



8 Maths Ncert Exemplar Chapter 7 2 Unit 7 Algebraic Expressions Identities And Factorisation A Main Concepts And Results I Algebraic Expression Terms Course Hero



Matrix Exponential Wikipedia



Rd Sharma Solutions For Class 8 Chapter 6 Algebraic Expressions And Identities Download Free Pdf


Evaluate 992 Using Suitable Identity Mathematics Topperlearning Com Gcgcpzlii
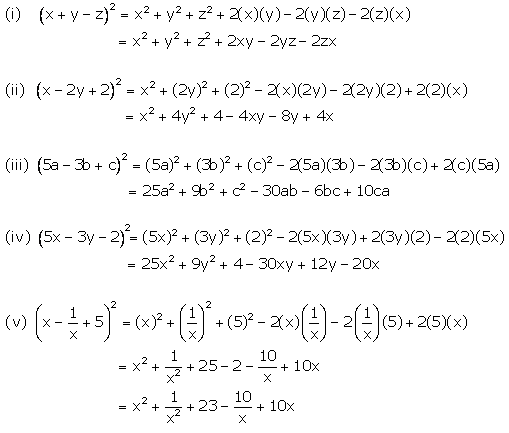


Chapter 4 Expansion Selina Concise Mathematics Part I Solutions For Class 9 Mathematics Icse Topperlearning



Expand Each Of The Following Using Suitable Identities Ii 2x Y Z Brainly In



Expand Each Of The Following Using Suitable Identities I


Expand Each Of The Following Using Suitable Identity I 2a 3b C 2 Sarthaks Econnect Largest Online Education Community


Lb6o6dgwjzwldm


Covid Economics Centre For Economic Policy Research



Expand Using Suitable Identities X 2y 4z 2 2 3a 7b C 2 Brainly In



Rise 9 X Whole Square Minus 12 X Minus Y Square Minus Z Cute766



Learning From Nature To Expand The Genetic Code Trends In Biotechnology



Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes



Ncert Exemplar Class 9 Maths Solutions Chapter 2 Polynomials Cbse Tuts



Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes



Cambridge International Examinations Cie Advanced Level Mathematics Pure Mathematics 2 3 By Ayman Alam Issuu



Expansions Popular Questions Icse Class 9 Math Mathematics Meritnation



Expansions Icse Class 9th Concise Selina Mathematics Icsehelp



Flowspec A Declarative Specification Language For Intra Procedural Flow Sensitive Data Flow Analysis Sciencedirect
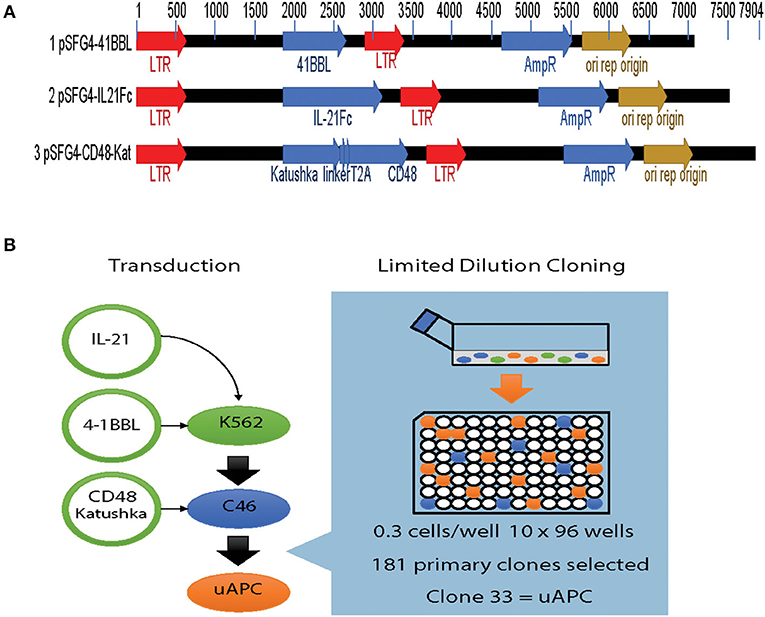


Frontiers Gmp Compliant Universal Antigen Presenting Cells Uapc Promote The Metabolic Fitness And Antitumor Activity Of Armored Cord Blood Car Nk Cells Immunology


Entropy Free Full Text Mesoscopic Simulation Of The 2 1 Dimensional Wave Equation With Nonlinear Damping And Source Terms Using The Lattice Boltzmann Bgk Model Html



Expand Each Of The Following Using Suitable Identities X 2y 4z 2 2x Y Z 2 2x 3y 2z 2 Youtube



Solve This Sum S Expand The Following Using Suitable Identity A 4x 2y A Heck Whether The Puiynomiai G X Maths Polynomials Meritnation Com



Expand Each Of The Following Using Suitable Identities I X 2y 4z 2 Ii 2x Y Z Youtube



Ma53 Tpde



Rna Toxicity In Non Coding Repeat Expansion Disorders The Embo Journal



Expand Each Of The Following Using Suitable Identities I X 2y 4z 2 Ii 2x Y Z 2 Ii Youtube



Ex 2 5 1 Class 9 Polynomials Use Suitable Identities To Find
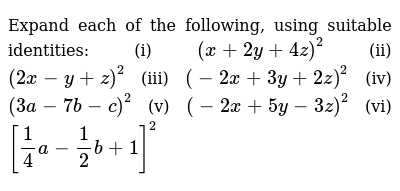


Expand The Following X 2y 4z 2



Cambridge International Examinations Cie Advanced Level Mathematics Pure Mathematics 2 3 By Ayman Alam Issuu



Expand Using The Identity A 2x 3y Z 2 Brainly In



Expand Using Suitable Identity 2x 5y 3z 2


Expand Each Of The Following Using Suitable Identities I X 2y 4z 2 Sarthaks Econnect Largest Online Education Community


Pplato Flap Math 3 3 Demoivre S Theorem And Complex Algebra


How To Use Python Lambda Functions Real Python



An Organoid Derived Bronchioalveolar Model For Sars Cov 2 Infection Of Human Alveolar Type Ii Like Cells The Embo Journal


Expand 1 X Y 3 Whole Cube Studyrankersonline



Complex Number Wikipedia



Ex 2 5 4 Expand Each Of The Following Using Suitable


Find 2x Y 3z 4x2 Y2 9z2 2xy 3yz 6xz Studyrankersonline



Expand Each Of The Following Using Suitable Identities 2x 3y 2z 2


Mathematical Programming Formulations For Piecewise Polynomial Functions Springerlink



Expanding Binomials Video Polynomials Khan Academy



Ex 2 5 4 Expand Each Of The Following Using Suitable



If Math X Y Z 0 Math What Is The Value Of Math Frac X 2 Yz Frac Y 2 Zx Frac Z 2 Xy Math Quora



Using Suitable Identities Expand The Following I X5 3y 2 Ii 11x 0 2y 2 Iii 4a 5b 2 Iv Y 25x 3 V 3a 5b 4c 2 Vi 12a B 13c 2



Expand Using Suitable Identity X 2y 4z 2 Brainly In



Ncert Class 9 Maths Lab Manual Verify The Algebraic Identity A B C A B C 2ab 2bc 2ca Cbse Tuts



Mathematics Fraction Mathematics Mathematical Objects



Crystal Structures Of Tdsc A Dibenzothiophene Monooxygenase From The Thermophile Paenibacillus Sp A11 2 Reveal Potential For Expanding Its Substrate Selectivity Journal Of Biological Chemistry


Using Suitable Identity Evaluate The Following Studyrankersonline


Polynomials Ppt Video Online Download



Expand Using Identities 3x 5y 2



Expand Each Of The Following Using Suitable Identities I X 2y 4z Square Brainly In


Cbse 9 Math Ncert Solutions


Perform Symbolic Computations Matlab Simulink



Polynomials Ppt Video Online Download



Ncert Solutions For Class 9 Maths Chapter 2 Polynomials In Pdf



Organizational Identity Formation And Change Academy Of Management Annals



0 件のコメント:
コメントを投稿